Top Notch Tips About What Is A Smooth Distribution How To Make Double Line Graph In Excel
More precisely, consists in a collection of vector subspaces with the following property.
What is a smooth distribution. These need not be linearly indepe… Many operations which are defined on smooth functions with compact support can also be defined for distributions. I plotted a probability distribution plot by simply executing:
You have a uniform distribution from 1 to 10^7 but you have stratified that range into 3 sections with sample sizes 10^4, 10^5, and 10^7. It will be readily apparent if you take a random set of input logits and visualize it yourself. I have a problem for to understand the following example of a distribution in the lee's book of smooth manifolds:
It is desired to sample the. Import matplotlib as plt plt.plot(df['value'], df['prob']) in which it returned. In general, if is a linear map that is continuous with respect to the weak topology, then it is not always possible to extend to a map by classic extension theorems of topology or linear functional analysis.
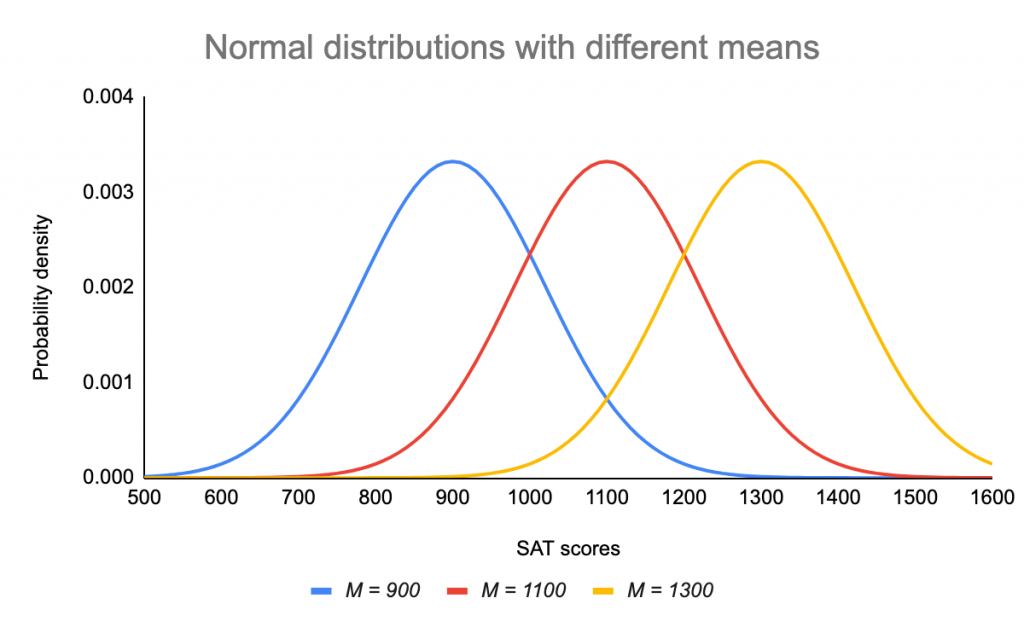
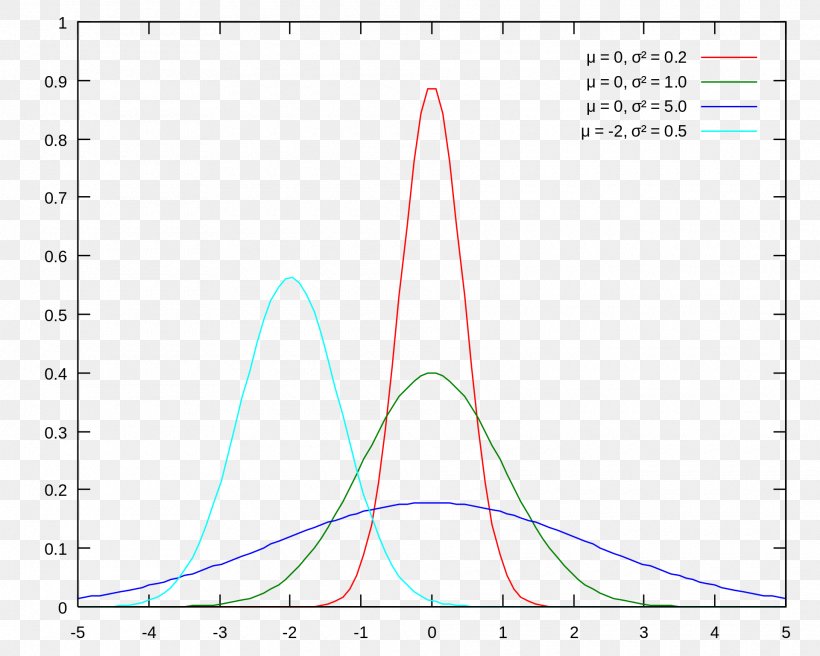
A (smooth) distribution assigns to any point a vector subspace in a smooth way. It means that the distribution is made less 'spiky', or accentuated. Let be a smooth manifold;
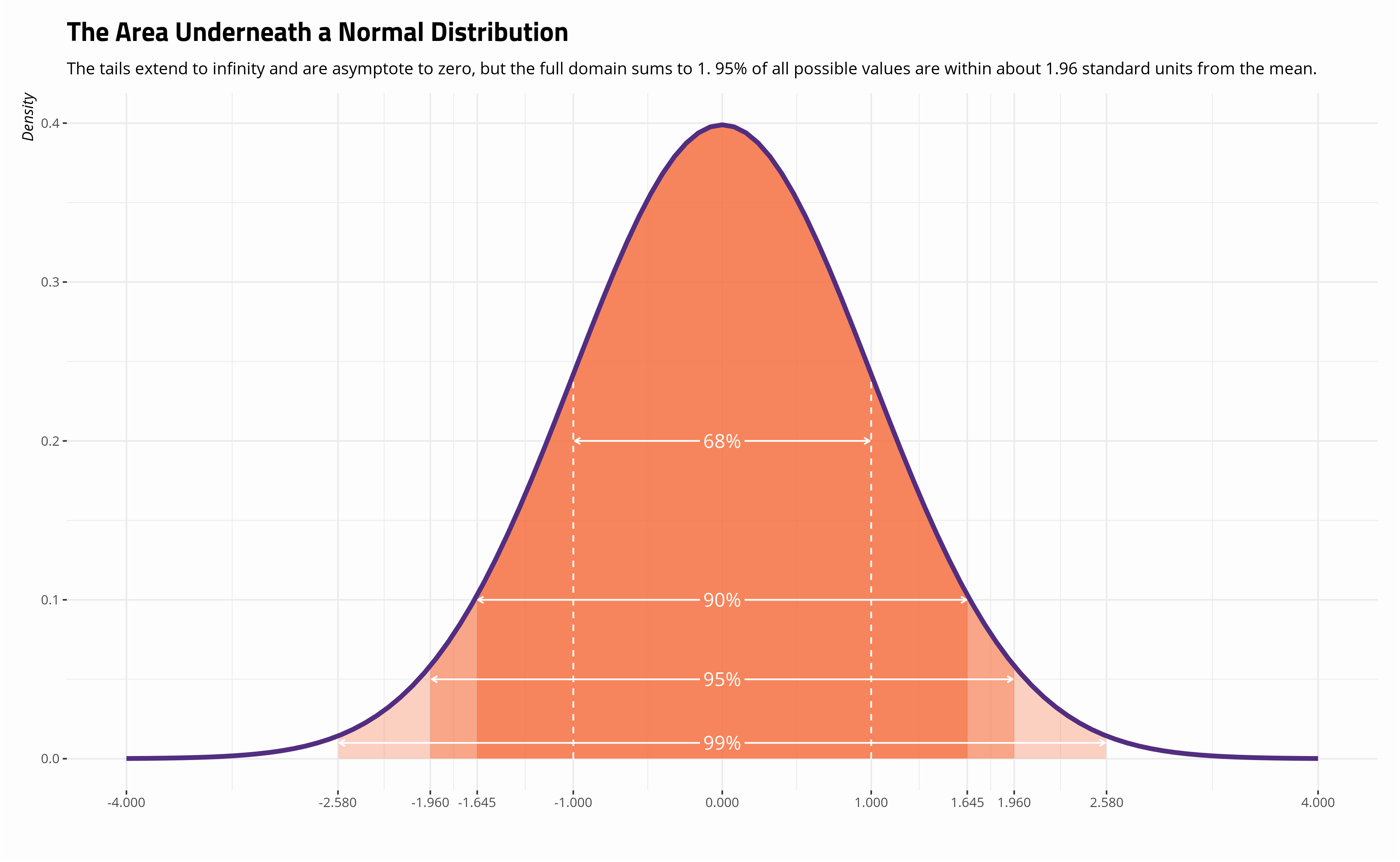
Around any there exist a neighbourhood and a collection of vector fields such that, for any point , span the set of smooth vector fields is also called a local basis of. In probability theory and statistics, smoothness of a density function is a measure which determines how many times the density function can be differentiated, or equivalently. Now if we assume for each point $p$ of $n$ there exist a neighborhood $u$ of $p$ and a set of smooth vector fields defined on $u$, denoted $\{ \tau_i \mid i \in i.
Suppose we have for all q ∈ u, an open set, δq = span(x ′ 1(q),., x ′ r(q)) for x ′ j ∈ x(n) ( c∞ vector. Since smoothness is a local property, we just need to show that, for every $x_0\in\mathbb{r}^3\setminus \mathrm{supp}(f)$, there is a neighborhood of $x_0$ on. Now, i would like to smooth the.
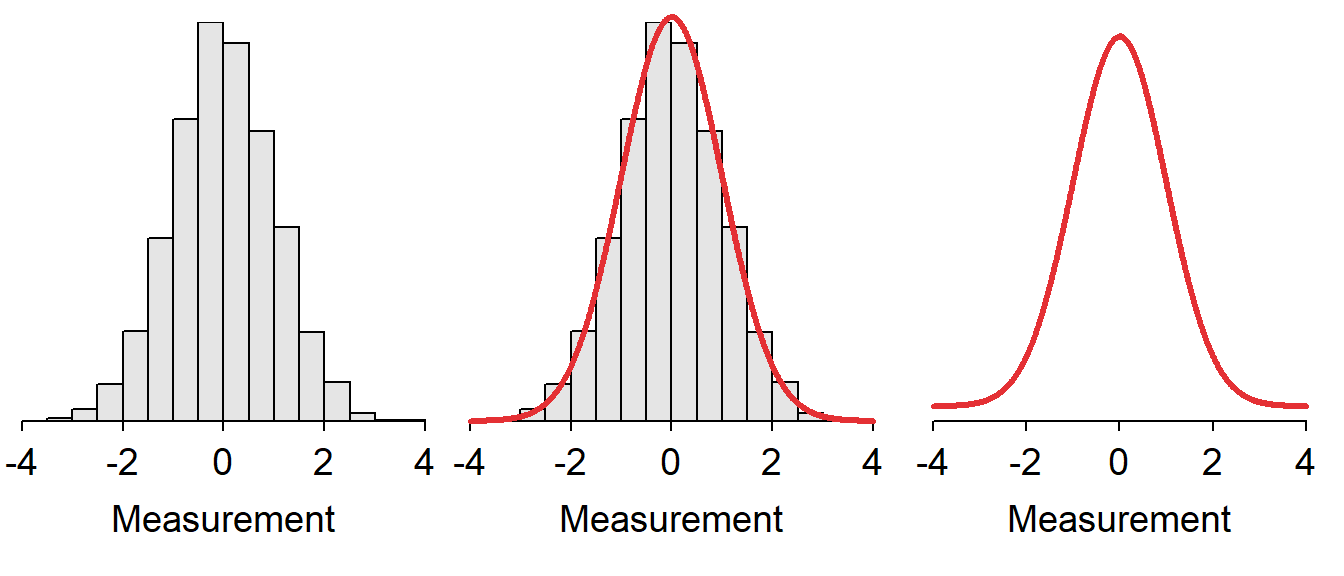
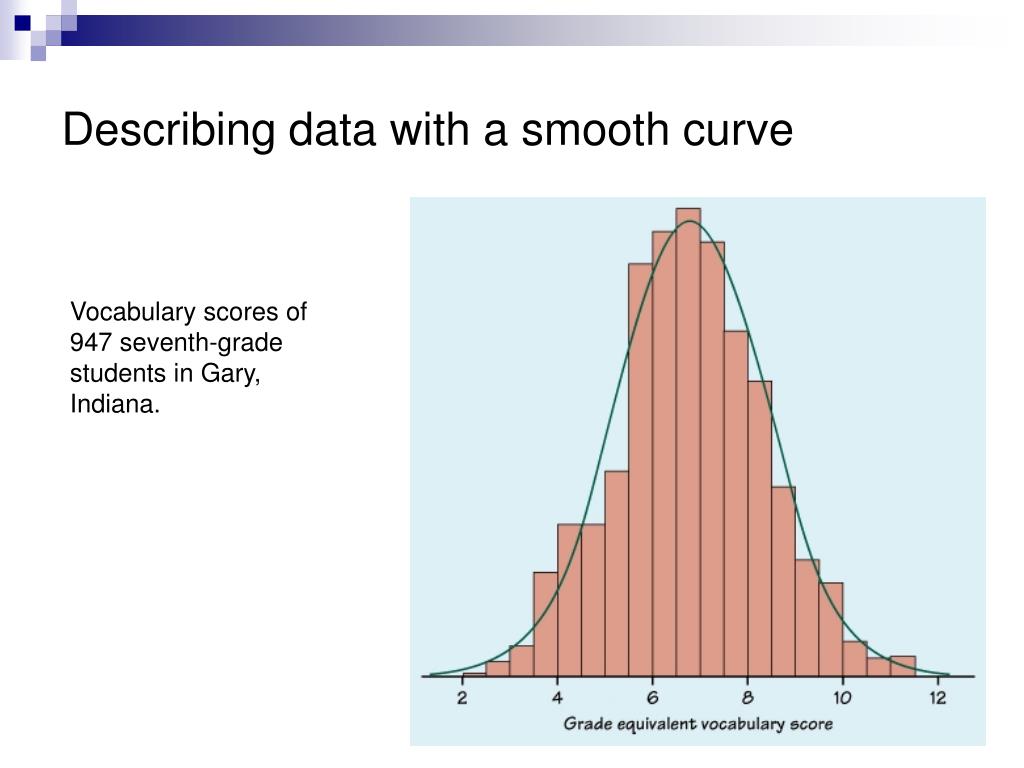
See also smooth function. In differential geometry, a smooth distribution is a set of vector fields that vary smoothly from point to point on a manifold (a curve or surface in higher dimensional space). A smoothed frequency curve is a smoothed curve, which is a graphic representation of the frequency distribution.
In statistics and image processing, to smooth a data set is to create an approximating function that attempts to capture important patterns in the data, while. The “distributional” extension of the above linear continuous operator a is possible if and only if a admits a schwartz adjoint, that is another linear continuous operator b o… I've reached an impasse in reading some texts on distribution theory, as several of them mention smooth distributions, but none of them actually define what it.
Let n be a smooth manifold. What is a smoothed frequency curve in statistics? A subbundle of variable dimension inside the tangent bundle of a smooth manifold is called a smooth distribution if it is the pointwise span of a family of smooth.
Let δ be a c∞ distribution on n.





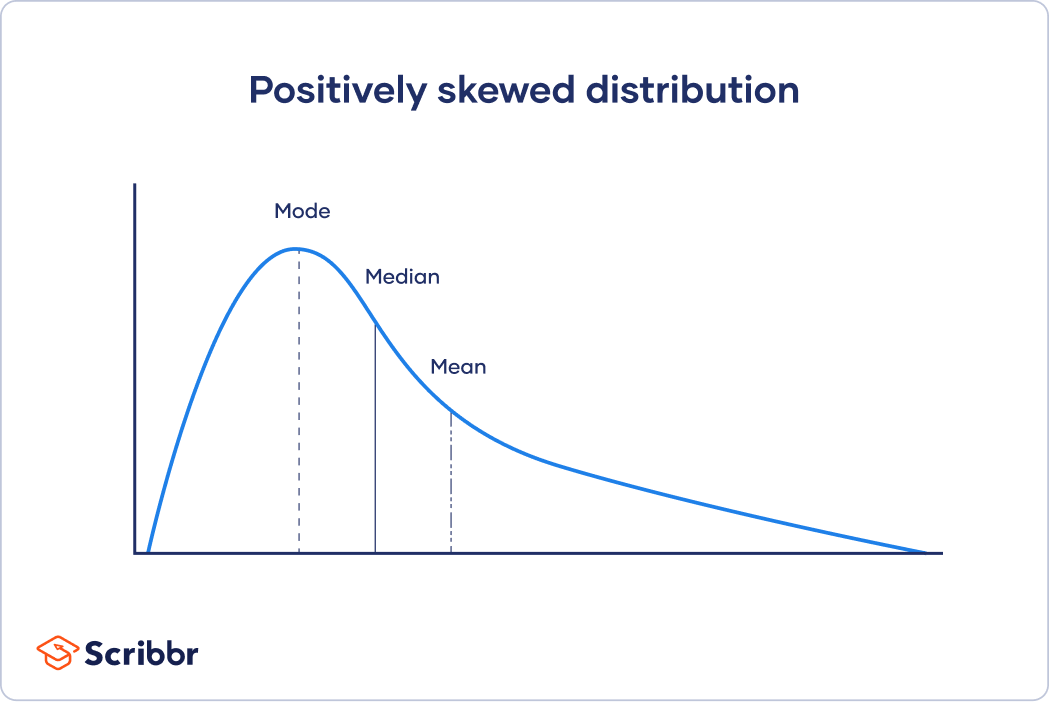
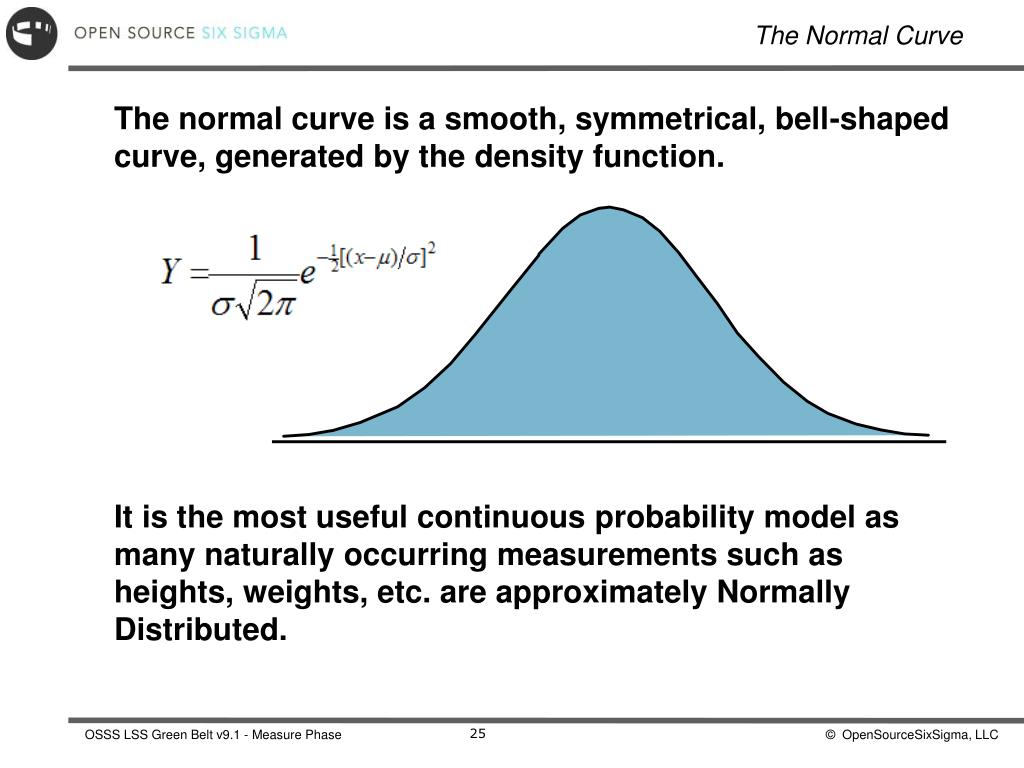






:max_bytes(150000):strip_icc()/dotdash_Final_Log_Normal_Distribution_Nov_2020-01-fa015519559f4b128fef786c51841fb9.jpg)